Niveau Maths sup

démonstration !

Posté par
eaty 24-03-08 à 23:23

Bonsoir à tous!

Pouvez-vous démontrer l'équivalence suivante ?

Pour toute fonction numérique g, définie au voisinage de x₀:

(dérivable en x₀, de nombre dérivé l)(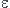>0,>0,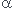(h,k)₊², 0<h+k $\frac{g(x_0+h)-g(x_0-k)}{h+k}-l$ <)

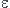merci à tous !

Posté par re : démonstration ! 25-03-08 à 01:23

Bonjour,

il manque certainement des choses à ton énoncé:

1)Des valeurs absolues

2)Le rôle de alpha

3)Tes recherches personnelles sur la question.

A bon entendeur...

Posté par re : démonstration ! 25-03-08 à 14:53

Bonjour ;

Je crois que l'énoncé proposé par eaty est :

$\fbox{\lim_{h\to0}\frac{g(x_0+h)-g(x_0)}{h}=\ell\in\mathbb{R}}\;\Longleftrightarrow\;\fbox{(\forall\varepsilon>0)\;(\exists\alpha>0)\;/\;(\forall h,k\in\mathbb{R})\;,\;0<h+k<\alpha\;\Longrightarrow\;\left|\frac{g(x_0+h)-g(x_0-k)}{h+k}-\ell\right|\;<\;\varepsilon}$ .

$\fbox{\Longleftarrow}$ En prenant $k=0$ on a $\fbox{(\forall\varepsilon>0)\;(\exists\alpha>0)\;/\;(\forall h\in\mathbb{R})\;,\;0<h<\alpha\;\Longrightarrow\;\left|\frac{g(x_0+h)-g(x_0)}{h}-\ell\right|\;<\;\varepsilon}$ $(1)$

En prenant $h=0$ on a $\fbox{(\forall\varepsilon>0)\;(\exists\alpha>0)\;/\;(\forall k\in\mathbb{R})\;,\;0<k<\alpha\;\Longrightarrow\;\left|\frac{g(x_0)-g(x_0-k)}{k}-\ell\right|\;<\;\varepsilon}$

en prenant , dans cette dernière expression $h=-k$ on a $\fbox{(\forall\varepsilon>0)\;(\exists\alpha>0)\;/\;(\forall h\in\mathbb{R})\;,\;-\alpha<h<0\;\Longrightarrow\;\left|\frac{g(x_0)-g(x_0+h)}{-h}-\ell\right|\;<\;\varepsilon}$

c'est à dire $\fbox{(\forall\varepsilon>0)\;(\exists\alpha>0)\;/\;(\forall h\in\mathbb{R})\;,\;-\alpha<h<0\;\Longrightarrow\;\left|\frac{g(x_0+h)-g(x_0)}{h}-\ell\right|\;<\;\varepsilon}$ $(2)$

et en combinant $(1)$ et $(2)$ on a le résultat.

$\fbox{\Longrightarrow}$ En écrivant $\fbox{\frac{g(x_0+h)-g(x_0-k)}{h+k}=\frac{h}{h+k}\frac{g(x_0+h)-g(x_0)}{h}+\frac{k}{h+k}\frac{g(x_0)-g(x_0-k)}{k}}$ on voit que si les deux nombres ,

$\frac{g(x_0+h)-g(x_0)}{h}$ et $\frac{g(x_0-k)-g(x_0)}{-k}$ , sont dans le convexe $[\ell-\varepsilon,\ell+\varepsilon]$ il en sera de même pour leur barycentre (à coefficients positifs). _{(sauf erreur bien entendu)}

Posté par re : démonstration ! 25-03-08 à 15:01

Bonjour
...et on continue à jouer à "je devine l'énoncé et je donne la solution"

Posté par re : démonstration ! 25-03-08 à 18:58

Excusez moi pour l'oubli de la valeur absolue...

J'ai pas fait les convexes encore . Puis je quand même conclure ?

Ce topic

Imprimer
Réduire / Agrandir
Pour plus d'options, connectez vous !
Fiches de maths

analyse en post-bac
21 fiches de mathématiques sur "analyse" en post-bac disponibles.

Rechercher

Espace membre

Zone membre

Pas de compte ?
Je m'inscris

Changer d'île

Cours et Exercices de maths

Forum de maths

college

lycee

Outils de maths

Pages les plus populaires

démonstration !

Seuls les membres peuvent poster sur le forum !

Ce topic

Fiches de maths